“the kelvin”

-as of [9 APRIL 2024]–

*abbreviated ‘K’*

(named after ‘william thomson’)

(1st baron kelvin)

(1824 – 1907)

*lived to ‘age 83’*

*then he simply ‘caught a chill’ and promptly perished*

(“as dignified as einstein”)

(of the ‘gentile kind’)

(“be gentle!” says emma)

*born in ‘belfast’ (/ ‘northern ireland’)*
(professor of ‘natural philosophy’ @ ‘university of glasgow’ for 53 years)

*the ‘kelvin’ is (1 / 273.16) of the ‘triple point’ of ‘water’*

(the “triple point” of a ‘substance’ is the ‘temperature’ + ‘pressure’ at which the 3 ‘phases’ of a ‘substance’ – [‘gas’ / ‘liquid’ / ‘solid’] – co-exist in ‘thermo-dynamic equilibrium’)

The kelvin is the base unit of temperature in the International System of Units (SI), having the unit symbol K.

The kelvin is now defined by fixing the numerical value of the ‘boltzmann constant k’ to 1.380 649×10−23 J⋅K−1.

The Boltzmann constant (kB or k) is the proportionality factor that relates the average relative kinetic energy of particles in a gas with the thermodynamic temperature of the gas

It occurs in the definitions of the kelvin and the gas constant, and in Planck’s law of black-body radiation and Boltzmann’s entropy formula.

The Boltzmann constant has the dimension energy divided by temperature, the same as entropy.

It is named after the Austrian scientist Ludwig Boltzmann.

As part of the 2019 redefinition of SI base units, the Boltzmann constant is one of the seven “defining constants” that have been given exact definitions.

They are used in various combinations to define the seven SI base units.

The Boltzmann constant is defined to be exactly 1.380649×10−23 J⋅K−1

Roles of the Boltzmann constant

Macroscopically, the ideal gas law states that, for an ideal gas, the product of pressure p and volume V is proportional to the product of amount of substance n (in moles) and absolute temperature T:

{\displaystyle pV=nRT,}
where R is the gas constant (8.31446261815324 J⋅K−1⋅mol−1).[4] Introducing the Boltzmann constant transforms the ideal gas law into an alternative form:

pV=NkT,
where N is the number of molecules of gas. For n = 1 mol, N is equal to the number of particles in one mole (the Avogadro number).

Role in the equipartition of energy[edit]
Given a thermodynamic system at an absolute temperature T, the average thermal energy carried by each microscopic degree of freedom in the system is 1/2kT (i.e., about 2.07×10−21 J, or 0.013 eV, at room temperature).

In classical statistical mechanics, this average is predicted to hold exactly for homogeneous ideal gases.

Monatomic ideal gases (the six noble gases) possess three degrees of freedom per atom, corresponding to the three spatial directions, which means a thermal energy of 3/2kT per atom. This corresponds very well with experimental data. The thermal energy can be used to calculate the root-mean-square speed of the atoms, which turns out to be inversely proportional to the square root of the atomic mass.

The root mean square speeds found at room temperature accurately reflect this, ranging from 1370 m/s for helium, down to 240 m/s for xenon.

Kinetic theory gives the average pressure p for an ideal gas as

p={\frac {1}{3}}{\frac {N}{V}}m{\overline {v^{2}}}.
Combination with the ideal gas law

pV=NkT
shows that the average translational kinetic energy is

{\tfrac {1}{2}}m{\overline {v^{2}}}={\tfrac {3}{2}}kT.
Considering that the translational motion velocity vector v has three degrees of freedom (one for each dimension) gives the average energy per degree of freedom equal to one third of that, i.e. 1/2kT.

The ideal gas equation is also obeyed closely by molecular gases; but the form for the heat capacity is more complicated, because the molecules possess additional internal degrees of freedom, as well as the three degrees of freedom for movement of the molecule as a whole. Diatomic gases, for example, possess a total of six degrees of simple freedom per molecule that are related to atomic motion (three translational, two rotational, and one vibrational). At lower temperatures, not all these degrees of freedom may fully participate in the gas heat capacity, due to quantum mechanical limits on the availability of excited states at the relevant thermal energy per molecule.

Role in Boltzmann factors[edit]
More generally, systems in equilibrium at temperature T have probability Pi of occupying a state i with energy E weighted by the corresponding Boltzmann factor:

P_{i}\propto {\frac {\exp \left(-{\frac {E}{kT}}\right)}{Z}},
where Z is the partition function. Again, it is the energy-like quantity kT that takes central importance.

Consequences of this include (in addition to the results for ideal gases above) the Arrhenius equation in chemical kinetics.

Role in the statistical definition of entropy[edit]

Boltzmann’s grave in the Zentralfriedhof, Vienna, with bust and entropy formula.

In statistical mechanics, the entropy S of an isolated system at thermodynamic equilibrium is defined as the natural logarithm of W, the number of distinct microscopic states available to the system given the macroscopic constraints (such as a fixed total energy E):

S=k\,\ln W.
This equation, which relates the microscopic details, or microstates, of the system (via W) to its macroscopic state (via the entropy S), is the central idea of statistical mechanics. Such is its importance that it is inscribed on Boltzmann’s tombstone.

The constant of proportionality k serves to make the statistical mechanical entropy equal to the classical thermodynamic entropy of Clausius:

\Delta S=\int {\frac {{\rm {d}}Q}{T}}.
One could choose instead a rescaled dimensionless entropy in microscopic terms such that

{S’=\ln W},\quad \Delta S’=\int {\frac {\mathrm {d} Q}{kT}}.
This is a more natural form and this rescaled entropy exactly corresponds to Shannon’s subsequent information entropy.

The characteristic energy kT is thus the energy required to increase the rescaled entropy by one nat.

The thermal voltage[edit]
In semiconductors, the Shockley diode equation—the relationship between the flow of electric current and the electrostatic potential across a p–n junction—depends on a characteristic voltage called the thermal voltage, denoted VT. The thermal voltage depends on absolute temperature T as

V_{\mathrm {T} }={kT \over q},
where q is the magnitude of the electrical charge on the electron with a value 1.602176634×10−19 C[5] Equivalently,

{\displaystyle {V_{\mathrm {T} } \over T}={k \over q}\approx 8.61733034\times 10^{-5}\ \mathrm {V/K} .}
At room temperature 300 K (27 °C; 80 °F), VT is approximately 25.85 mV.[6][7] and at the standard state temperature of 298.15 K (25.00 °C; 77.00 °F), it is approximately 25.69 mV. The thermal voltage is also important in plasmas and electrolyte solutions (e.g. the Nernst equation); in both cases it provides a measure of how much the spatial distribution of electrons or ions is affected by a boundary held at a fixed voltage

History

The Boltzmann constant is named after its 19th century Austrian discoverer, Ludwig Boltzmann.

Although Boltzmann first linked entropy and probability in 1877, the relation was never expressed with a specific constant until Max Planck first introduced k, and gave a precise value for it (1.346×10−23 J/K, about 2.5% lower than today’s figure), in his derivation of the law of ‘black body radiation’ in 1900–1901

Before 1900, equations involving Boltzmann factors were not written using the energies per molecule and the Boltzmann constant, but rather using a form of the gas constant R, and macroscopic energies for macroscopic quantities of the substance. The iconic terse form of the equation S = k ln W on Boltzmann’s tombstone is in fact due to Planck, not Boltzmann. Planck actually introduced it in the same work as his eponymous h.[11]

In 1920, Planck wrote in his Nobel Prize lecture:[12]

This constant is often referred to as Boltzmann’s constant, although, to my knowledge, Boltzmann himself never introduced it — a peculiar state of affairs, which can be explained by the fact that Boltzmann, as appears from his occasional utterances, never gave thought to the possibility of carrying out an exact measurement of the constant.

This “peculiar state of affairs” is illustrated by reference to one of the great scientific debates of the time. There was considerable disagreement in the second half of the nineteenth century as to whether atoms and molecules were real or whether they were simply a heuristic tool for solving problems. There was no agreement whether chemical molecules, as measured by atomic weights, were the same as physical molecules, as measured by kinetic theory. Planck’s 1920 lecture continued:[12]

Nothing can better illustrate the positive and hectic pace of progress which the art of experimenters has made over the past twenty years, than the fact that since that time, not only one, but a great number of methods have been discovered for measuring the mass of a molecule with practically the same accuracy as that attained for a planet.

In versions of SI prior to the 2019 redefinition of the SI base units, the Boltzmann constant was a measured quantity rather than a fixed value. Its exact definition also varied over the years due to redefinitions of the kelvin (see Kelvin § History) and other SI base units (see Joule § History).

In 2017, the most accurate measures of the Boltzmann constant were obtained by acoustic gas thermometry, which determines the speed of sound of a monatomic gas in a triaxial ellipsoid chamber using microwave and acoustic resonances.[13][14] This decade-long effort was undertaken with different techniques by several laboratories;[a] it is one of the cornerstones of the 2019 redefinition of SI base units. Based on these measurements, the CODATA recommended 1.380 649 × 10−23 J⋅K−1 to be the final fixed value of the Boltzmann constant to be used for the International System of Units.[15]

Value in different units[edit]
Values of k Units Comments
1.380649×10−23 J/K SI by definition, J/K = m2⋅kg/(s2⋅K) in SI base units
8.617333262×10−5 eV/K [note 1]
2.083661912×1010 Hz/K (k/h) [note 1]
1.380649×10−16 erg/K CGS system, 1 erg = 1×10−7 J
3.297623483×10−24 cal/K [note 1] 1 calorie = 4.1868 J
1.832013046×10−24 cal/°R [note 1]
5.657302466×10−24 ft lb/°R [note 1]
0.695034800 cm−1/K (k/(hc)) [note 1]
0.001985875 kcal/(mol⋅K) (kNA) [note 1]
0.008314463 kJ/(mol⋅K) (kNA) [note 1]
−228.5991672 dB(W/K/Hz) 10 log10(k/(1 W/K/Hz)),[note 1] used for thermal noise calculations
Since k is a proportionality factor between temperature and energy, its numerical value depends on the choice of units for energy and temperature. The small numerical value of the Boltzmann constant in SI units means a change in temperature by 1 K only changes a particle’s energy by a small amount. A change of 1 °C is defined to be the same as a change of 1 K. The characteristic energy kT is a term encountered in many physical relationships.

The Boltzmann constant sets up a relationship between wavelength and temperature (dividing hc/k by a wavelength gives a temperature) with one micrometer being related to 14387.777 K, and also a relationship between voltage and temperature (multiplying the voltage by k in units of eV/K) with one volt being related to 11604.518 K. The ratio of these two temperatures, 14387.777 K / 11604.518 K ≈ 1.239842, is the numerical value of hc in units of eV⋅μm.

Planck units[edit]
The Boltzmann constant provides a mapping from this characteristic microscopic energy E to the macroscopic temperature scale T = E/k. In physics research another definition is often encountered in setting k to unity, resulting in the Planck units or natural units for temperature and energy. In this context temperature is measured effectively in units of energy and the Boltzmann constant is not explicitly needed.[16]

The equipartition formula for the energy associated with each classical degree of freedom then becomes

{\displaystyle E_{\mathrm {dof} }={\tfrac {1}{2}}T\ }
The use of natural units simplifies many physical relationships; in this form the definition of thermodynamic entropy coincides with the form of information entropy:

S=-\sum P_{i}\ln P_{i}.
where Pi is the probability of each microstate.

The value chosen for a unit of the Planck temperature is that corresponding to the energy of the Planck mass.

See also[edit]
CODATA 2018
Thermodynamic beta
Notes[edit]
^ Independent techniques exploited: acoustic gas thermometry, dielectric constant gas thermometry, johnson noise thermometry. Involved laboratories cited by CODATA in 2017: LNE-Cnam (France), NPL (UK), INRIM (Italy), PTB (Germany), NIST (USA), NIM (China).
^ Jump up to: a b c d e f g h i The value is exact but not expressible as a finite decimal; approximated to 9 decimal places only.
References[edit]
^ The International System of Units (SI) (PDF) (9th ed.), Bureau International des Poids et Mesures, 2019, p. 129
^ Richard Feynman (1970). The Feynman Lectures on Physics Vol I. Addison Wesley Longman. ISBN 978-0-201-02115-8.
^ “2018 CODATA Value: Boltzmann constant”. The NIST Reference on Constants, Units, and Uncertainty. NIST. 20 May 2019. Retrieved 20 May 2019.
^ “Proceedings of the 106th meeting” (PDF). 16–20 October 2017.
^ “2018 CODATA Value: elementary charge”. The NIST Reference on Constants, Units, and Uncertainty. NIST. 20 May 2019. Retrieved 20 May 2019.
^ Rashid, Muhammad H. (2016). Microelectronic circuits: analysis and design (Third ed.). Cengage Learning. pp. 183–184. ISBN 9781305635166.
^ Cataldo, Enrico; Lieto, Alberto Di; Maccarrone, Francesco; Paffuti, Giampiero (18 August 2016). “Measurements and analysis of current-voltage characteristic of a pn diode for an undergraduate physics laboratory”. arXiv:1608.05638v1 [physics.ed-ph].
^ Kirby, Brian J. (2009). Micro- and Nanoscale Fluid Mechanics: Transport in Microfluidic Devices. Cambridge University Press. ISBN 978-0-521-11903-0.
^ Tabeling, Patrick (2006). Introduction to Microfluidics. Oxford University Press. ISBN 978-0-19-856864-3.
^ Planck, Max (1901), “Ueber das Gesetz der Energieverteilung im Normalspectrum” (PDF), Ann. Phys., 309 (3): 553–63, Bibcode:1901AnP…309..553P, doi:10.1002/andp.19013090310, archived from the original (PDF) on 10 June 2012. English translation: “On the Law of Distribution of Energy in the Normal Spectrum”. Archived from the original on 17 December 2008.
^ Duplantier, Bertrand (2005). “Le mouvement brownien, ‘divers et ondoyant'” Brownian motion, ‘diverse and undulating’. Séminaire Poincaré 1 (in French): 155–212.
^ Jump up to: a b Planck, Max (2 June 1920), The Genesis and Present State of Development of the Quantum Theory (Nobel Lecture)
^ Pitre, L; Sparasci, F; Risegari, L; Guianvarc’h, C; Martin, C; Himbert, M E; Plimmer, M D; Allard, A; Marty, B; Giuliano Albo, P A; Gao, B; Moldover, M R; Mehl, J B (1 December 2017). “New measurement of the Boltzmann constant by acoustic thermometry of helium-4 gas”. Metrologia. 54 (6): 856–873. Bibcode:2017Metro..54..856P. doi:10.1088/1681-7575/aa7bf5.
^ de Podesta, Michael; Mark, Darren F; Dymock, Ross C; Underwood, Robin; Bacquart, Thomas; Sutton, Gavin; Davidson, Stuart; Machin, Graham (1 October 2017). “Re-estimation of argon isotope ratios leading to a revised estimate of the Boltzmann constant” (PDF). Metrologia. 54 (5): 683–692. Bibcode:2017Metro..54..683D. doi:10.1088/1681-7575/aa7880.
^ Newell, D. B.; Cabiati, F.; Fischer, J.; Fujii, K.; Karshenboim, S. G.; Margolis, H. S.; Mirandés, E. de; Mohr, P. J.; Nez, F. (2018). “The CODATA 2017 values of h, e, k, and N A for the revision of the SI”. Metrologia. 55 (1): L13. Bibcode:2018Metro..55L..13N. doi:10.1088/1681-7575/aa950a. ISSN 0026-1394.
^ Kalinin, M; Kononogov, S (2005), “Boltzmann’s Constant, the Energy Meaning of Temperature, and Thermodynamic Irreversibility”, Measurement Techniques, 48 (7): 632–36, doi:10.1007/s11018-005-0195-9, S2CID 118726162
External links[edit]
Draft Chapter 2 for SI Brochure, following redefinitions of the base units (prepared by the Consultative Committee for Units)
Big step towards redefining the kelvin:

Scientists find new way to determine Boltzmann constant

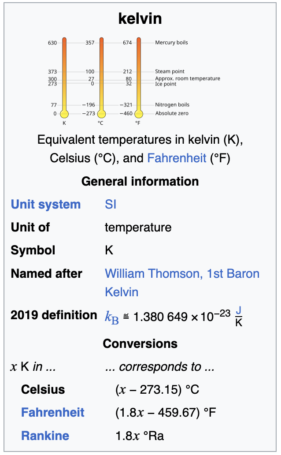
This unit is equal to kg⋅m2⋅s−2⋅K−1, where the kilogram, metre and second are defined in terms of the Planck constant, the speed of light, and the duration of the caesium-133 ground-state hyperfine transition respectively.

It is named after the Belfast-born Glasgow University engineer and physicist William Thomson, 1st Baron Kelvin (1824–1907).

Thus, this definition depends only on universal constants, and not on any physical artifacts as practiced previously, such as the International Prototype of the Kilogram, whose mass diverged over time from the original value.

One kelvin is equal to a change in the thermodynamic temperature T that results in a change of thermal energy kT by 1.380 649×10−23 J.[2]

The Kelvin scale fulfills Thomson’s requirements as an absolute thermodynamic temperature scale. It uses absolute zero as its null point.

Unlike the degree Fahrenheit and degree Celsius, the kelvin is not referred to or written as a degree. The kelvin is the primary unit of temperature measurement in the physical sciences, but is often used in conjunction with the degree Celsius, which has the same magnitude.

History[edit]

In 1848, William Thomson, who was later ennobled as Lord Kelvin, wrote in his paper On an Absolute Thermometric Scale of the need for a scale whereby “infinite cold” (absolute zero) was the scale’s null point, and which used the degree Celsius for its unit increment. Kelvin calculated that absolute zero was equivalent to −273 °C on the air thermometers of the time.[3] This absolute scale is known today as the Kelvin thermodynamic temperature scale. Kelvin’s value of “−273” was the negative reciprocal of 0.00366—the accepted expansion coefficient of gas per degree Celsius relative to the ice point, giving a remarkable consistency to the currently accepted value.

In 1954, Resolution 3 of the 10th General Conference on Weights and Measures (CGPM) gave the Kelvin scale its modern definition by designating the triple point of water as its second defining point and assigned its temperature to exactly 273.16 kelvin.[4]

In 1967/1968, Resolution 3 of the 13th CGPM renamed the unit increment of thermodynamic temperature “kelvin”, symbol K, replacing “degree Kelvin”, symbol °K.[5] Furthermore, feeling it useful to more explicitly define the magnitude of the unit increment, the 13th CGPM also held in Resolution 4 that “The kelvin, unit of thermodynamic temperature, is equal to the fraction 1/273.16 of the thermodynamic temperature of the triple point of water.”[6]

In 2005, the Comité International des Poids et Mesures (CIPM), a committee of the CGPM, affirmed that for the purposes of delineating the temperature of the triple point of water, the definition of the Kelvin thermodynamic temperature scale would refer to water having an isotopic composition specified as Vienna Standard Mean Ocean Water.[7]

On 16 November 2018, a new definition was adopted, in terms of a fixed value of the Boltzmann constant. With this change the triple point of water became an empirically determined value of approximately 273.16 kelvin. For legal metrology purposes, the new definition officially came into force on 20 May 2019, the 144th anniversary of the Metre Convention.[8]

Usage conventions[edit]
According to the International Bureau of Weights and Measures, when spelled out or spoken, the unit is pluralised using the same grammatical rules as for other SI units such as the volt or ohm (e.g. “… the triple point of water is exactly 273.16 kelvins”[9]). When reference is made to the “Kelvin scale”, the word “kelvin”—which is normally a noun—functions adjectivally to modify the noun “scale” and is capitalized. As with most other SI unit symbols (angle symbols, e.g. 45° 3′ 4″, are the exception) there is a space between the numeric value and the kelvin symbol (e.g. “99.987 K”).[10][11] (The style guide for CERN, however, specifically says to always use “kelvin”, even when plural.)[12]

Before the 13th CGPM in 1967–1968, the unit kelvin was called a “degree”, the same as with the other temperature scales at the time. It was distinguished from the other scales with either the adjective suffix “Kelvin” (“degree Kelvin”) or with “absolute” (“degree absolute”) and its symbol was °K.

The latter term (degree absolute), which was the unit’s official name from 1948 until 1954, was ambiguous since it could also be interpreted as referring to the Rankine scale.

Before the 13th CGPM, the plural form was “degrees absolute”.

The 13th CGPM changed the unit name to simply “kelvin” (symbol: K).[13]

The omission of “degree” indicates that it is not relative to an arbitrary reference point like the Celsius and Fahrenheit scales (although the Rankine scale continued to use “degree Rankine”), but rather an absolute unit of measure which can be manipulated algebraically

(e.g. multiplied by two to indicate twice the amount of “mean energy” available among elementary degrees of freedom of the system)

2019 redefinition[edit]
In 2005 the CIPM embarked on a programme to redefine the kelvin (along with the other SI units) using a more experimentally rigorous methodology. In particular, the committee proposed redefining the kelvin such that Boltzmann constant takes the exact value 1.3806505×10−23 J/K.[14] The committee had hoped that the programme would be completed in time for its adoption by the CGPM at its 2011 meeting, but at the 2011 meeting the decision was postponed to the 2014 meeting when it would be considered as part of a larger programme.[15]

The redefinition was further postponed in 2014, pending more accurate measurements of Boltzmann’s constant in terms of the current definition,[16] but was finally adopted at the 26th CGPM in late 2018, with a value of k = 1.380649×10−23 J/K.[14][17]

From a scientific point of view, the main advantage is that this will allow measurements at very low and very high temperatures to be made more accurately, as the techniques used depend on the Boltzmann constant. It also has the philosophical advantage of being independent of any particular substance. The challenge was to avoid degrading the accuracy of measurements close to the triple point. From a practical point of view, the redefinition will pass unnoticed; water will still freeze at 273.15 K (0 °C),[18] and the triple point of water will continue to be a commonly used laboratory reference temperature.

The difference is that, before the redefinition, the triple point of water was exact and the Boltzmann constant had a measured value of 1.38064903(51)×10−23 J/K, with a relative standard uncertainty of 3.7×10−7.[17] Afterward, the Boltzmann constant is exact and the uncertainty is transferred to the triple point of water, which is now 273.1600(1) K.

Practical uses[edit]

Kelvin temperature conversion formulae

from kelvins to kelvins

Celsius [°C] = [K] − 273.15 [K] = [°C] + 273.15

Fahrenheit [°F] = [K] × 9⁄5 − 459.67 [K] = ([°F] + 459.67) × 5⁄9

Rankine [°R] = [K] × 9⁄5 [K] = [°R] × 5⁄9

For temperature intervals rather than specific temperatures,

1 K = 1 °C = 9⁄5 °F = 9⁄5 °R

Comparisons among various temperature scales

Colour temperature

The kelvin is often used as a measure of the colour temperature of light sources.

Colour temperature is based upon the principle that a ‘black body radiator’ emits light with a frequency distribution characteristic of its temperature.

Black bodies at temperatures below about 4000 K appear reddish, whereas those above about 7500 K appear bluish. Colour temperature is important in the fields of image projection and photography, where a colour temperature of approximately 5600 K is required to match “daylight” film emulsions. In astronomy, the stellar classification of stars and their place on the Hertzsprung–Russell diagram are based, in part, upon their surface temperature, known as effective temperature. The photosphere of the Sun, for instance, has an effective temperature of 5778 K.

Digital cameras and photographic software often use colour temperature in K in edit and setup menus. The simple guide is that higher colour temperature produces an image with enhanced white and blue hues. The reduction in colour temperature produces an image more dominated by reddish, “warmer” colours.

Kelvin as a unit of noise temperature[edit]
In electronics, the kelvin is used as an indicator of how noisy a circuit is in relation to an ultimate noise floor, i.e. the noise temperature. The so-called Johnson–Nyquist noise of discrete resistors and capacitors is a type of thermal noise derived from the Boltzmann constant and can be used to determine the noise temperature of a circuit using the Friis formulas for noise.

Unicode character[edit]
The symbol is encoded in Unicode at code point U+212A K KELVIN SIGN. However, this is a compatibility character provided for compatibility with legacy encodings. The Unicode standard recommends using U+004B K LATIN CAPITAL LETTER K instead; that is, a normal capital K. “Three letterlike symbols have been given canonical equivalence to regular letters: U+2126 Ω OHM SIGN, U+212A K KELVIN SIGN, and U+212B Å ANGSTROM SIGN. In all three instances, the regular letter should be used.”[19]

☯✨✨✨✨✨✨✨✨✨✨✨✨✨✨✨✨✨🚀THE 2ND NATURE🚀

1 Trackback / Pingback