“release me…”
.
.
.
.
.
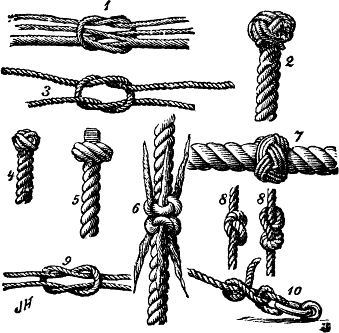
.
1 – ‘yarn not’
.
2 – ‘manrope knot’
.
3 – ‘granny knot’
(“care to try?)
(i’d rather not)
.
4 – ‘wall + crown knot’
.
5 – ‘matthew walker’s knot’
(i want a knot name after me too!)
(mom!!)
.
6 – ‘shroud knot’
.
7 – ‘turk’s head knot’
.
.
*UNTIE ME!*
*LOCK ‘EM UP!”
‘LOCK’ + ‘LOAD’*
(“AND GET READY FOR SOME GOOD OLE…”…)
.
-in ‘mathematics’, a (k)not is an ’embedding’ of a ‘circle’ in ‘3-dimensional euclidean space’ – R3 (also known as E3) – considered up to ‘continuous deformations’ (‘isotopies’)-
.
(a crucial difference between the standard ‘mathematical’ + ‘conventional’ notions of a ‘knot’ is that ‘mathematical knots’ are ‘closed’—there are no ‘ends’ to ‘tie’ or ‘untie’ on a ‘mathematical knot’)
(‘physical properties’ such as ‘friction’ + ‘thickness’ also do not apply, although there are ‘mathematical definitions’ of a ‘knot’ that take such properties into account)
(the term knot is also applied to embeddings of Sj(Sn) in Sn(Sn), especially in the case j=n−2j = n – 2)
.
(the branch of ‘mathematics’ that studies ‘knots’ is known as ‘knot theory’, and has many simple relations to ‘graph theory’)
.
.
“WHY DO HEADPHONES GET SPONTANEOUSLY KNOTTED?”
“THE INDEPENDENT”
(BRITISH NEWSPAPER)
*LINK*
.
*THE STUDY*
(‘dorian raymer’ + ‘douglas smith’)
.
.
[the reason that any ‘string’ gets tangled so easily depends on 2 factors…]
“critical string length”
“agitation time”
.
Researchers investigated earphone tangles the only way they could – but putting different lengths of cable in a box and ‘agitating’ them repeatedly
.
It was probably the 21st century’s preeminent sage Bill Murray that summed up the problem most succinctly:
“How to tie the strongest knot ever:
1) Put some headphones in your pocket
2) Wait one minute”
.
But have you ever wondered exactly why this happens?
Well, scientists have and to find out what mysterious pocket-sized-goings-on are going on they did what any sensible researcher would:
they put different pieces of wire in a box and shook them up to see if they got knotted.
They did this a lot, in fact, 3,415 times in all.
The results (detailed in a now quite venerable 2006 paper entitled “Spontaneous knotting of an agitated string” by Dorian M. Raymer and Douglas E. Smith) showed that two key factors cause complex knots to “form within seconds”: “critical string length” and “agitation time”
.
The probability of knot forming versus string length.
The probability of knot forming versus string length
.
Essentially the longer the lengths of cable and the more they’re shaken the more likely it is that a knot will spontaneously form
Qualities such as cable rigidity and diameter also play a part but it’s length and time spent tumbling that matter most – and unfortunately, these are the factors you’re least likely to change.
Raymer and Smith came to their conclusion by experimenting with cables of varying length and different “agitation times,” finding that cables shorter than 46 centimetres will rarely – if ever – get knotted, with the probability increasing with cable size before plateauing just after 2 metres in length.
For the average pair of earphones (defined as 139 centimetres in length) this means the probability of a knot spontaneously forming in an enclosed space about the size of your bag is just under 50 per cent.
That’s right, every time you dump your earphones after arriving at school or work there’s a one in two chance you’re dicing with death going to be slightly annoyed later in the day.
Knot formation in action.
The box on the left is the rotating container used by Raymer and Smith, with one crossed wire quickly leading to chaos.
Knot formation in action.
The box on the left is the rotating container used by Raymer and Smith, with one crossed wire quickly leading to chaos.
Raymer and Smith also noted that the Y shape of earphones increases the chance of knotting substantially, with only one end of the wire or cable having to cross another to start off the tumble-weed reaction of a spontaneous tangle.
All in all, the pair identified 120 different types of knot in their trials, including “all prime knots with up to seven crossings”.
Next time you drag a sorry clump of cables from your pocket just be glad you weren’t assisting on that particular un-tangling
.
Register for free to continue reading
Registration is a free and easy way to support our truly independent journalism
By registering, you will also enjoy limited access to Premium articles, exclusive newsletters, commenting, and virtual events with our leading journalists
Please enter a valid email
Please enter a valid email
Password
Must be at least 6 characters, include an upper and lower case character and a number
Must be at least 6 characters, include an upper and lower case character and a number
Must be at least 6 characters, include an upper and lower case character and a number
First name
Please enter your first name
Special characters aren’t allowed
Please enter a name between 1 and 40 characters
Last name
Please enter your last name
Special characters aren’t allowed
Please enter a name between 1 and 40 characters
You must be over 18 years old to register
You must be over 18 years old to register
Year of birth
I would like to be emailed about offers, events and updates from The Independent.
Read our Privacy notice
You can opt-out at any time by signing in to your account to manage your preferences. Each email has a link to unsubscribe.
Already have an account? sign in
www.independent.co.uk /news/science/why-do-your-earphones-get-tangled-in-your-pocket-science-has-the-answer-9548540.html
Why do your earphones get tangled in your pocket? Science has the answer
James Vincent4-5 minutes
6/19/2014
NewsScience
.
.
.
.
.
.
.
.
.
.
.



 *SKETCHES*
*SKETCHES*

 *
*
 |/\-*WIKI-LINK*-/\|
|/\-*WIKI-LINK*-/\|



















 *
*