.
*aka ‘negative one’*
*aka ‘zero‘*
*aka ‘one’*
*aka ‘two’*
*aka ‘three’*
*aka ‘four’*
*aka ‘five’*
*aka ‘six’*
*aka ‘seven’*
*aka ‘eight’*
*aka ‘nine’*
*aka ‘ten’*
*aka ‘eleven’*
*aka ‘twelve’*
.
*13*
*aka ‘thirteen’*
*14*
*aka ‘fourteen’*
*15*
*aka ‘fifteen’*
*aka ‘sixteen’*
.
.
.
.
.
.
*a number is a ‘mathematical object’ used to [‘count’ / ‘measure’ / ‘label’]*
.
(the original examples are the ‘natural numbers’ 1, 2, 3, 4 and so forth)
(a ‘notational symbol’ that represents a ‘number’ is called a ‘numeral’)
(in addition to their use in ‘counting’ and ‘measuring’, ‘numerals’ are often used for ‘labels’ (as with ‘telephone numbers’), for ‘ordering’ (as with ‘serial numbers’), and for ‘codes’ (as with ISBNs))
(in ‘common usage’, number may refer to a ‘symbol’, a ‘word’, or a ‘mathematical abstraction’)
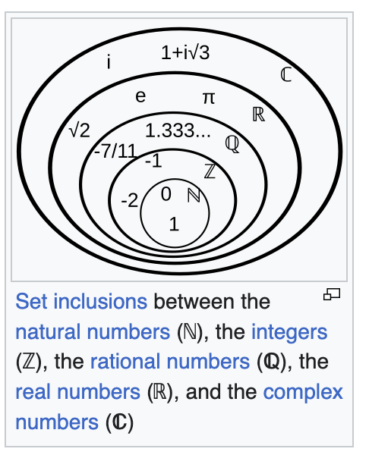
(in ‘mathematics’, the notion of ‘number’ has been extended over the ‘centuries’ to include ‘0’, ‘negative numbers’, ‘rational numbers’ (such as 1/2 and β2/3), ‘real numbers’ (such as β2 and Ο), and ‘complex numbers’, which extend the ‘real numbers’ by adding a ‘square root’ of ‘β1′)
(calculations with ‘numbers’ are done with ‘arithmetical operations’, the most familiar being ‘addition’, ‘subtraction’, ‘multiplication’, ‘division’, and ‘exponentiation’)
(their ‘study’ or ‘usage’ is called ‘arithmetic’)
(the same term may also refer to ‘number theory’, the study of the ‘properties’ of ‘numbers’)
(besides their ‘practical uses’, ‘numbers’ have ‘cultural significance’ throughout the ‘world’)
(for example, in ‘western society’, the number ’13’ is regarded as ‘unlucky’, and “a million” may signify “a lot”)
(though it is now regarded as ‘pseudoscience’, ‘numerology’, the belief in a ‘mystical significance’ of ‘numbers’, permeated ‘ancient’ and ‘medieval’ thought)
(“numerology” heavily influenced the development of ‘greek mathematics’, stimulating the ‘investigation’ of many ‘problems’ in ‘number theory’ which are still ‘of interest’ today)
(during the ’19th century’, ‘mathematicians’ began to develop many different ‘abstractions’ which share certain ‘properties’ of ‘numbers’ and may be seen as extending the ‘concept’)
(among the first were the ‘hypercomplex numbers’, which consist of various ‘extensions’ or ‘modifications’ of the ‘complex number system’)
.
(today, ‘number systems’ are considered important special examples of much more general categories such as ‘rings’ + ‘fields’, and the application of the term “number” is a matter of ‘convention’, without ‘fundamental significance’)
.
.
.
.
πππβ*βMATHEMATICSβ* β πππ
.
.
πππππ€ππ€ππ€ππ€β€οΈπππ§‘β£οΈπππβ£οΈπ§‘ππβ€οΈπ€ππ€ππ€ππ€ππππ
.
.
*πβ¨ *TABLE OF CONTENTS* β¨π·*
.
.
π₯π₯π₯π₯π₯π₯*we won the war* π₯π₯π₯π₯π₯π₯