VII
SEVEN
.
*he 4th ‘prime number’*
is not only a Mersenne prime (since 23 − 1 = 7)
…but also a double Mersenne prime since the exponent, 3, is itself a ‘mersenne prime’)
.
(it is also a ‘double mersenne prime
Newman–Shanks–Williams prime,
a Woodall prime,
a factorial prime,
a lucky prime,
a happy number (happy prime),
a safe prime (the only Mersenne safe prime),
and the fourth Heegner number)
.
“o get over yourself!”
“the embittered old man”
.
“you’ll see…”
“the man with a plan”
.
“soon you’ll be sorry”
.
(‘seven’ is the lowest natural number that cannot be represented as the sum of the squares of 3 integers)
.
*lagrange’s 4-square theorem*
(and its ‘historical development’)
Seven is the aliquot sum of one number, the cubic number 8
…and is the base of the 7-aliquot tree.
n = 7 is the first natural number for which the next statement does not hold:
“Two nilpotent endomorphisms from Cn with the same minimal polynomial and the same rank are similar.”
7 is the only number D for which the equation 2n − D = x2 has more than two solutions for n and x natural. In particular, the equation 2n − 7 = x2 is known as the Ramanujan–Nagell equation.
7 is the only dimension, besides the familiar 3, in which a vector cross product can be defined.
7 is the lowest dimension of a known exotic sphere, although there may exist as yet unknown exotic smooth structures on the 4-dimensional sphere.
999,999 divided by 7 is exactly 142,857. Therefore, when a vulgar fraction with 7 in the denominator is converted to a decimal expansion, the result has the same six-digit repeating sequence after the decimal point, but the sequence can start with any of those six digits.For example, 1/7 = 0.142857 142857… and 2/7 = 0.285714 285714….
In fact, if one sorts the digits in the number 142857 in ascending order,
124578, it is possible to know from which of the digits the decimal part of the number is going to begin with.
(the remainder of dividing any number by ‘7’ will give the position in the sequence ‘124578 that the decimal part of the resulting number will start.
For example, 628 ÷ 7 = 89 5/7; here 5 is the remainder, and would correspond to number 7 in the ranking of the ascending sequence.
So in this case, 628 ÷ 7 = 89.714285.
Another example, 5238 ÷ 7 = 748 2/7, hence the remainder is 2, and this corresponds to number 2 in the sequence. In this case, 5238 ÷ 7 = 748.285714.
A seven-sided shape is a heptagon. The regular n-gons for n ≤ 6 can be constructed by compass and straightedge alone, but the regular heptagon cannot. Figurate numbers representing heptagons (including seven) are called heptagonal numbers. Seven is also a centered hexagonal number.
Seven is the first integer reciprocal (multiplicative inverse) with infinitely repeating sexagesimal representation.
There are seven frieze groups, the groups consisting of symmetries of the plane whose group of translations is isomorphic to the group of integers.
.
*there are 7 fundamental types of catastrophes*
.
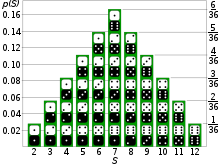
(graph of the probability distribution of the sum of 2 six-sided dice)
.
When rolling two standard six-sided dice, seven has a 6 in 36 (or 1/6) probability of being rolled (1–6, 6–1, 2–5, 5–2, 3–4, or 4–3), the greatest of any number.
(the ‘millennium prize problems’ are 7 problems in mathematics that were stated by the ‘clay mathematics institute’ in ‘2000’)
(currently, 6 of the problems remain unsolved)
.
.
.
.
.
.
.
.
.
.
.
.
.





















 *
*